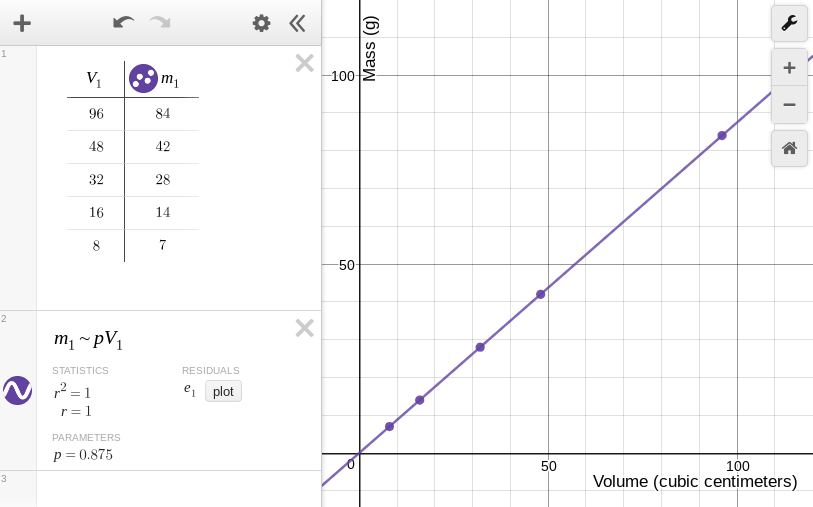
- __________ _____ Determine the density ρ of the soap based on the data and graph.
- ______________ Will the soap float or sink?
- ______________ Is this soap likely to be [Jergens/Ivory] OR [Dial] soap?
- __________ _____ Calculate the mass of a bar of the soap that has a volume of 80 cm³.
- __________ _____ Calculate the volume of a bar of the soap that has a mass of 78.75 grams.
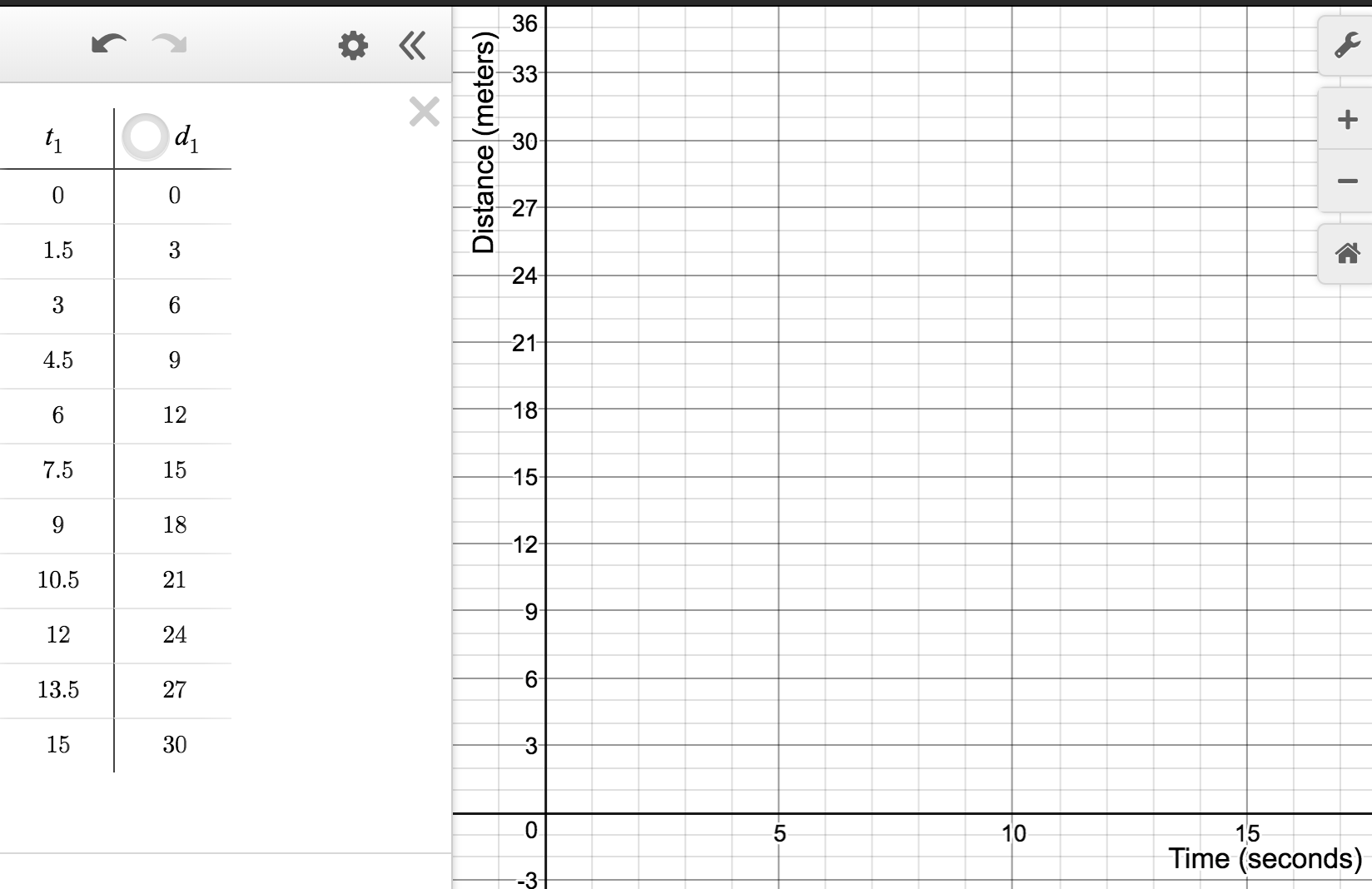
- Use the data to plot the line on the graph. Note that the data is linear.
- __________ __________ Calculate the velocity of the RipStik.

- __________ __________ What is the experimentally measured acceleration of gravity?
- __________ __________ What is the raw error with respect to the published value of 9.8 m/s²?
- ________________ What is the percent error?
- ______________________ How good is the agreement of the experimental value to the published value?
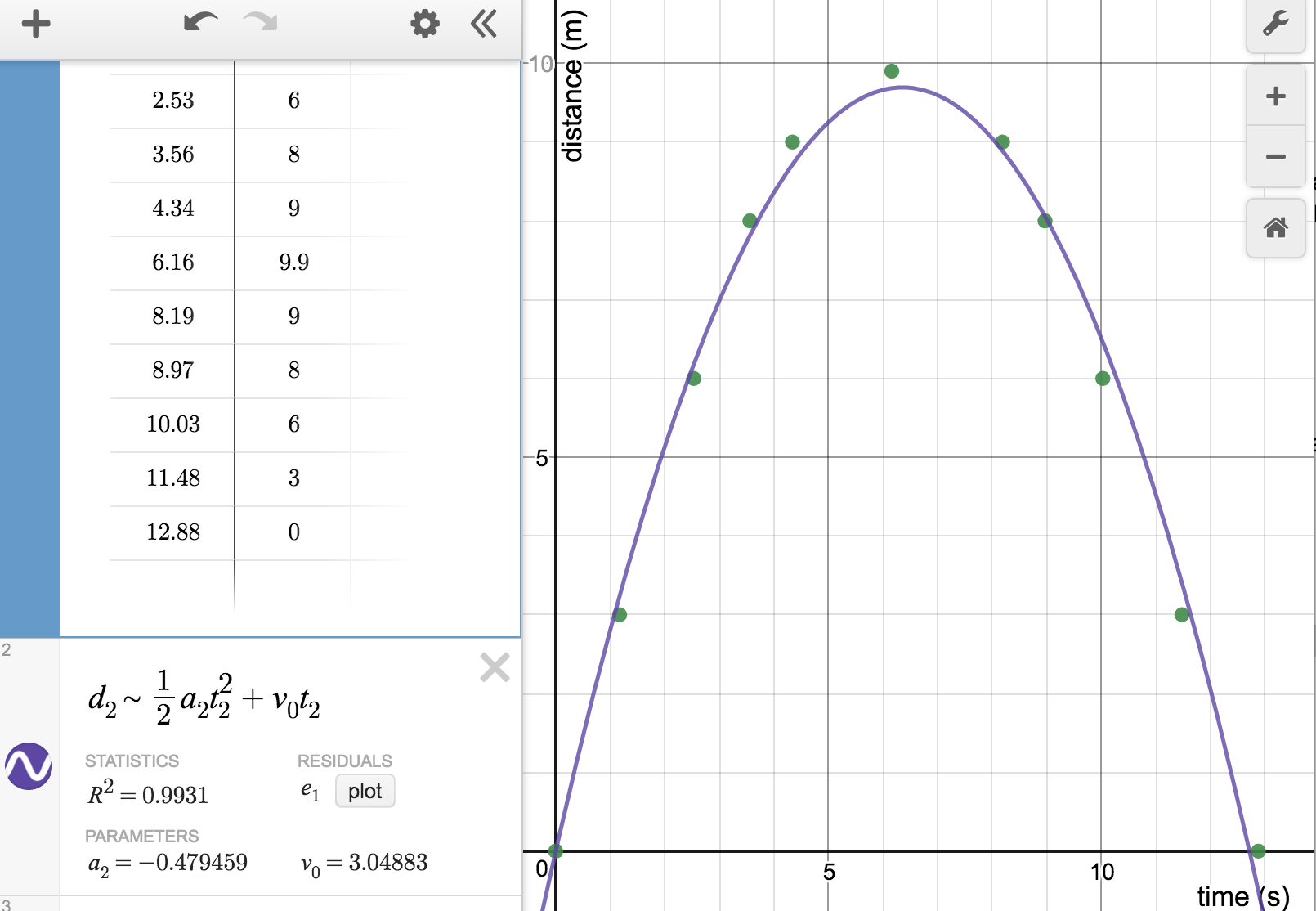
- acceleration a₂: __ __________ __________
- initial velocity v₀: __________ __________
- __________ __________ After a time t₂ of eleven seconds, what will be the distance d₂ of the RipStik?
- __ __________ __________ After a time t₂ of twenty seconds, what will be the distance d₂ of the RipStik?
Explain the conservation of momentum as demonstrated in experiments with marbles during the fourth week.
Why?
A -5 ℃
B 0 ℃
C 12 ℃
D 29 ℃
E 37 ℃
F 47 ℃
G 100 ℃
B 0 ℃
C 12 ℃
D 29 ℃
E 37 ℃
F 47 ℃
G 100 ℃
- ____ inside air temperature/room temperature Pohnpei during daytime
- ____ asphalt road temperature on a sunny day
- ____ boiling water
- ____ freezing water
- ____ human body core temperature
- ____ lower refrigerator section of a refrigerator
- ____ upper freezer section of a refrigerator
- _______________ To go from the faculty office to where Lee Ling was hiding, did you mostly have to go North, south, east, or west?
- _________ _____ Lee Ling was hiding at N 6° 54.678'. Calculate the difference in arcminutes between the south faculty office and Lee Ling.
- _________ _____Use the difference in arc minutes from part a) and the published value of 1843 meters per arcminute of latitude here on Pohnpei to estimate the distance in meters you had to walk to get to Lee Ling's location.