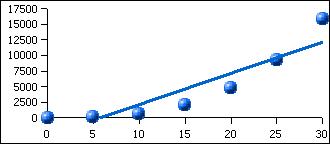
| Wind speed (mph) | Power (Watts) | 
|
||||||
| 0 | 0 | |||||||
| 5 | 75 | |||||||
| 10 | 588 | |||||||
| 15 | 1980 | |||||||
| 20 | 4700 | |||||||
| 25 | 9190 | |||||||
| 30 | 15876 | |||||||
| 1 | 499.79 | Calculate the slope of the linear regression (trend line) for the data. | ||||||
| 2 | -2866.93 | Calculate the y-intercept of the linear regression (trend line) for the data. | ||||||
| 3 | positive | Is the correlation positive or negative? | ||||||
| 4 | 17124.5 | Calculate the projected power production in Watts for a wind speed of 40 mph. | ||||||
| 5 | 7.74 | Calculate the wind speed for which power production is 1000 Watts. | ||||||
| 6 | 0.9064 | Determine the correlation coefficient r. | ||||||
| 7 | high or strong | Is the correlation none, low, moderate, high, or perfect? | ||||||
| 8 | non-linear | Does the relationship appear to be linear or non-linear? | ||||||
| 9 | 0.8216 | Determine the coefficient of determination. | ||||||
| 10 | 82.16% | What percent in the var in wind speed accounts for the var in the kilowatt hours? | ||||||
| 11 | Yes | In this case, is there causation? | ||||||
| [ans will vary] | Why or why not? | |||||||