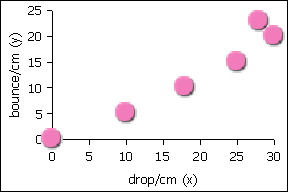

| Drop/cm (x) | Bounce/cm (y) |
|---|---|
| 0 | 0 |
| 10 | 5 |
| 18 | 10 |
| 25 | 15 |
| 28 | 23 |
| 30 | 20 |
| Linear Regression Functions | |||
|---|---|---|---|
| Statistic or Parameter | Math symbol | Stat symbol | OpenOffice |
| Slope | m | b | =slope(y-data;x-data) |
| Intercept | b | a | =intercept(y-data;x-data) |
| Correlation | r | =correl(y-data;x-data) | |
| Coefficient of Determination | r2 | =(correl(y-data;x-data))^2 | |