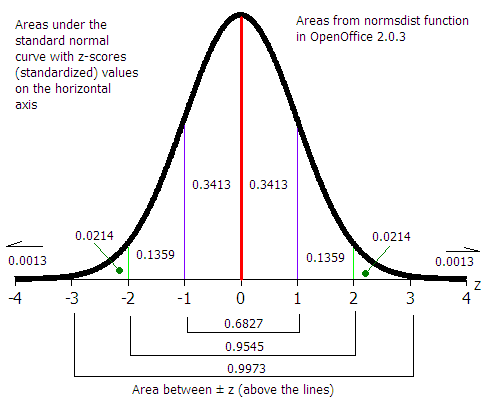
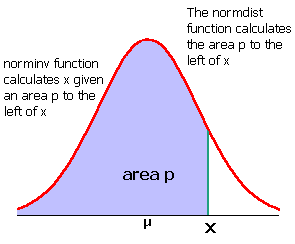
MS 150 Statistics Test 4 • Name:

In preparation for the upcoming half marathon on Saturday 19 July I am averaging μ = 9.24 kilometers per hour with a standard deviation σ = 0.70 kilometers per hour. Use these numbers in the following calculations. Presume that my speeds are normally distributed.

1. ______________ If my speed falls below 8.4 kilometers per hour, then the duration of my run will exceed my endurance limit. In other words, at speeds less than 8.4 kilometers per hour I will be moving so slowly that I will exceed the two and half hours I am able to run. Use the normal distribution to determine the probability that my speed will be less than 8.4 kilometers per hour.
2. ______________ If my speed is faster than 11 kilometers per hour I am likely to win prize money. Calculate the probability that my speed will exceed 11 kilometers per hour.
3. ______________ What is the speed below which are the 20% slowest speeds? This number is useful as it tells me when I performing significantly below par. Nota bene: This is an area p to x problem!
4. ______________ When I am training I track my speed in real time using a GPS unit. To optimize my training my speed should remain in the upper 33% of my speed range. Calculate the speed above which (faster than) is 33% of the area under the normal curve. This speed is the minimum speed I should strive to hold while training.
5. ______________ Thursday evening 26 June 2008 was hot, sunny, and humid - the worst possible conditions for a half. Dehydration, heat exhaustion, heat stroke, or hyponatremia are all risk factors. The half-marathon is usually run at 4:00. If the day of the half is like that of Thursday evening, then the half will be both difficult and potentially dangerous. To acclimatize to the heat, I went out for a run under the sun. I ran 12.2 km, about7.7 miles at an easy pace of 8.76 kilometers per hour (kph). What percent of my runs are between 8.76 kph and 9.24 kph?