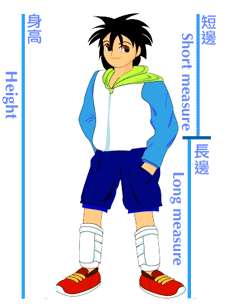

Calculate your FiboBelly ratio;
Divide your height by your belly button height to get your FiboBelly ratio.
Put your FiboBelly ratio on the board!
The sides of the boxes in order are 1, 1, 2, 3, 5, 8, 13, 21,... Do you see the pattern? Do you see how to get the next number? What are the next five FiboBox numbers?
_____ _____ _____ _____ _____
Add up the widths of the boxes across the top: 1 + 1 + 3 + 8 + 21 = ______
Did you get a FiboBox number?
Add up the widths of the boxes down the left side: 1 + 2 + 5 + 13 = ______
Did you get a FiboBox number?
Calculate the ratios of the sides of the rectangles.
Do at home: Use a calculator to find the following FiboRatios.
Find the next four FiboRatios using the next four FiboBox numbers from above:
Is the Fibobox ratio (FiboRatio) close to the average FiboBelly ratio?
The theory is that the ratio of successive sides of FiboBoxes, also known as Fibonacci squares, converge ("meet") at a special number called the "golden ratio." The golden ratio is what I have been calling the FiboRatio. The FiboRatio is actually called the Fibonacci Ratio. The following mathematical reasoning generates the exact value of the golden ratio.
The Fibonacci ratio is
Note that the smaller rectangle on the left will also have the same Fibonacci ratio for the long side divided by the short side:
Therefore
As the rectangles can be any arbitrary side length, I can decide that y = 1 and calculate the value of x.
The solution to the above quadratic is:
Is the same as the Fibonacci ratio further above? Use a calculator!