MS 150 Statistics Summer 2001 Quiz Four: Sect 9.4

Name: _______________________________

In math courses at the national campus Spring 2001 the campuswide population mean grade point average (GPA) m was 1.613.  During the Spring 2001 term at the national campus 15 Yapese male students attained a sample mean GPA of 1.133 with a standard deviation sx of 1.407 in math courses.  At an alpha a of 0.1, is the Yapese male math GPA statistically significantly lower than the national campus math GPA?

1. What is Ho? 
   
   
2. What is H₁?
   
   
3. What is alpha?
   
   
4. Is this a one-tailed or a two-tailed test?
   
   
5. What is the value of n?
   
   
6. How many degrees of freedom are there?
   
   
7. Calculate the t-statistic for the data.
   
   
   
   
8. What is the t_c critical value?
   
   
   
   
9. Make a sketch of the student's t-distribution curve including the critical value for t, the critical area, and the t-statistic.  Use the back if necessary to making a clean, clear, concise, legible, and accurate sketch.
   
   
   
   
   
   
10. Do we reject Ho or do we fail to reject Ho?
    
    
    
11. Is the Yapese male student GPA statistically significantly below the campuswide math GPA at an alpha of 0.1?
    
    
    

Statistic	Equations	Excel
Square root		=SQRT(number)
Sample size	n	=COUNT(data)
Sample mean		=AVERAGE(data)
Population mean	m x P(x) n p (binomial)	=AVERAGE(data)
Sample standard deviation	sx	=STDEV(data)
Population standard deviation	s (binomial)	=STDEVP(data)
Slope		=SLOPE(y data, x data)
Intercept		=INTERCEPT(y data, x data)
Correlation		=CORREL(y data, x data)
Binomial probability	= nCr pr q(n-r)	=COMBIN(n,r)*p^r*q^(n-r)
Calculate a z value from an x	z =	=STANDARDIZE(x, m, s)
Calculate an x value from a z	x = s z + m
Calculate a z value from an 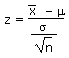value given m and s		=STANDARDIZE(x, m, s/SQRT(n))
Find a probability p from a z value		=NORMSDIST(z)
Find a z value from a probability p		=NORMSINV(p)
Standard error of the population mean
Standard error of the sample mean
Determining z critical zc from a for confidence intervals or two-tail tests.		=NORMSINV(1-a/2)
Error tolerance E of a mean for n ³ 30 using s		=CONFIDENCE(a,s,n)
Error tolerance E of a mean for n ³ 30 using sx	E =	=CONFIDENCE(a,sx,n)
Error tolerance E of a mean for n < 30.  Can also be used for n ³ 30.		[no Excel function, determine tc and then multiply by standard error of the mean as shown in the equation]
Determining tc from a and the degrees of freedom df for a confidence interval or a two-tail test.		=TINV(a,df)
Calculate an value from a tc	=+ m
Calculate a confidence interval for a population mean m from a sample mean and an error tolerance E	-E< m <+E
Determining zc from a for a two-tail hypothesis test.		=NORMSINV(a/2) [returns only the negative value for zc]
Determining zc from a for a one-tail hypothesis test.		=NORMSINV(a) [returns the right tail zc, change the sign for the left tail]
Determining tc from a and degrees of freedom df for a two-tail hypothesis test.		=TINV(a, df) [returns only the positive value for tc]
Determining tc from a and degrees of freedom df for a one-tail hypothesis test.		=TINV(2a, df) [returns only the left-tail tc, change the sign for right-tail]
Determining the one-tail p-value for a z-statistic z for a negative value of z		=NORMSDIST(z)
Determining the one-tail p-value for a z-statistic z for a positive value of z		=NORMSDIST(1-z)
Determining the two-tail p-value for the absolute value of the z-statistic		=2*NORMSDIST(1-|z|)
Determining the one-tail p-value for a t-statistic t and degrees of freedom df		=TDIST(t,df,1) [TDIST accepts only positive values for t, use the absolute value of t]
Determining the two-tail p-value for a t-statistic t and degrees of freedom df		=TDIST(t,df,2)

Statistic home Lee Ling home COM-FSM home page