 Color can be described in many different ways.
There are color words such as red, orange, yellow, green, and blue.
In optical physics each color is described by a wavelength and frequency of light.
Color can be described in many different ways.
There are color words such as red, orange, yellow, green, and blue.
In optical physics each color is described by a wavelength and frequency of light.
| Hue name | Hue angle° |
|---|---|
| red | 9 |
| orange-red | 17 |
| red-orange | 24 |
| red-orange | 24 |
| dark orange | 26 |
| orange | 30 |
| yellow-orange | 36 |
| orange-yellow | 43 |
| goldenrod | 48 |
| gold | 52 |
| yellow | 64 |
| green-yellow | 77 |
| chartreuse | 88 |
| green | 111 |
| mint | 137 |
| aquamarine | 165 |
| cyan | 184 |
| deep sky blue | 200 |
| royal blue | 217 |
| blue | 230 |
| blue | 236 |
| blue | 239 |
| indigo | 250 |
| purple | 270 |
| magenta | 303 |
 Color can be described in many different ways.
There are color words such as red, orange, yellow, green, and blue.
In optical physics each color is described by a wavelength and frequency of light.
Color can be described in many different ways.
There are color words such as red, orange, yellow, green, and blue.
In optical physics each color is described by a wavelength and frequency of light.
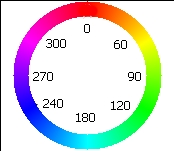
A third way to describe color is by using a color wheel or circle. The word hue is used for the word color when working with a color wheel. On a color wheel the colors are arranged in a circle in the same order that the colors appear in a rainbow. While in art class one learns that the primary colors for paint are red, yellow, and blue; the primary colors for light are different. The primary colors of light are red, green, and blue.
Red, the first color in the rainbow, is placed at the top and is assigned a color angle (or hue angle) of 0°. Once around a circle is 360°. Green is placed one third of the way around the circle at 120°. Blue is placed two-thirds of the way around the circle at 240°.
In the first section the image of a rainbow was analyzed from red to purple, with the hue angles being recorded at equal distances across the face of the rainbow.
The web page uses Cascading Style Sheets 3 Color Module HSL color values and requires the use of a browser that supports CSS3 such as Firefox.
For the hue angle data given in the table:
| Bins | Frequency | Relative Frequency f/n |
|---|---|---|
| _________ | _________ | _________ |
| _________ | _________ | _________ |
| _________ | _________ | _________ |
| _________ | _________ | _________ |
| _________ | _________ | _________ |
| _________ | _________ | _________ |
| Sums: | _________ | _________ |
Construct a 95% confidence interval for the population mean µ hue angle for a rainbow. Note that n is less than 30. Use the sample size n, sample mean x and sample standard deviation sx from questions two, three, and nine above to generate your t-critical tc and error tolerance E.
On a color wheel or circle, the mean hue angle is cyan at 180° degrees. Test the hypothesis that the population mean µ hue angle for a rainbow is 180° degrees, in other words, that the middle of the rainbow is actually cyan. Use 180° for the population mean µ. Use the sample size n, sample mean x and sample standard deviation sx from questions two, three, and nine. Use an alpha of α = 0.05 for the hypothesis test.
| Pixel | Hue angle° |
|---|---|
| 0 | 9 |
| 15 | 17 |
| 30 | 24 |
| 45 | 24 |
| 60 | 26 |
| 75 | 30 |
| 90 | 36 |
| 105 | 43 |
| 120 | 48 |
| 135 | 52 |
| 150 | 64 |
| 165 | 77 |
| 180 | 88 |
| 195 | 111 |
| 210 | 137 |
| 225 | 165 |
| 240 | 184 |
| 255 | 200 |
| 270 | 217 |
| 285 | 230 |
| 300 | 236 |
| 315 | 239 |
| 330 | 250 |
| 345 | 270 |
| 360 | 303 |
This section explores whether the hue angle changes linearly as one moves from red to magenta across a rainbow. The image of a rainbow was analyzed from red to purple. The hue angle for every 15th picture element (pixel) was measured and recorded.
| Basic Statistics | |||
|---|---|---|---|
| Statistic or Parameter | Symbol | Equations | Excel |
| Square root | =SQRT(number) | ||
| Sample standard deviation | sx or s | =STDEV(data) | |
| Sample Coefficient of Variation | CV | sx/x | =STDEV(data)/AVERAGE(data) |
| Confidence interval statistics | |||
|---|---|---|---|
| Statistic or Parameter | Symbol | Equations | Excel |
| Degrees of freedom | df | = n-1 | =COUNT(data)-1 |
| Find a zc value from a confidence level c | zc | =ABS(NORMSINV((1-c)/2)) | |
| Find a tc value from a confidence level c | tc | =TINV(1-c,df) | |
| Calculate an error tolerance E of a mean for n < 30 using sx. Should also be used for n ≥ 30. | E | =tc*sx/SQRT(n) | |
| Calculate a confidence interval for a population mean µ from a sample mean x and an error tolerance E | x-E≤ µ ≤x+E | ||
| Hypothesis Testing | |||
| Calculate t-critical for a two-tailed test | tc | =TINV(α,df) | |
| Calculate a t-statistic | t |  |
=(x - µ)/(sx/SQRT(n)) |
| Calculate a two-tailed p-value from a t-statistic | p | = TDIST(ABS(t),df,2) | |
| Linear Regression Statistics | |||
|---|---|---|---|
| Statistic or Parameter | Symbol | Equations | Excel |
| Slope | b | =SLOPE(y data, x data) | |
| Intercept | a | =INTERCEPT(y data, x data) | |
| Correlation | r | =CORREL(y data, x data) | |
| Coefficient of Determination | r2 | =(CORREL(y data, x data))^2 | |
| Statistics | fx |
|---|---|
| Count | 55 |
| min | 14 |
| max | 47 |
| range | 33 |
| mode | 37 |
| median | 36 |
| mean-stdv | 26.06 |
| mean | 34.11 |
| mean+stdv | 42.16 |
| mean/max | 0.73 |
| stdev | 8.05 |
| cv | 0.24 |
| curve mx | 50.19 |
| 1 | 47 |
| 0.9 | 42.3 |
| 0.8 | 37.6 |
| 0.7 | 32.9 |
| 0.6 | 28.2 |
Score distribution on the final examination:
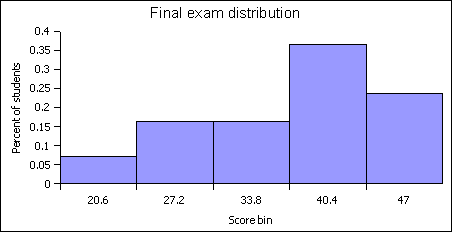
Grade distribution on final examination only:
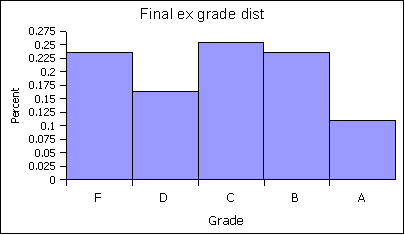
Grade distribution for the course: