Test 02 | 7.1 to 9.2 • Name:

Chapter Seven: Normal curve problems
Song mahs
x: cars/5 min
7
2
12
5
19
7
13
7
4
6
16
10

The data is the number of cars per five minute period passing past Song Mahs, Pehleng, Kitti over the course of an hour from 17:08 to 18:08 on Monday evening, 30 October 2006.

  1. x = ______________ Calculate the sample mean x number of cars per five minute period.
  2. sx = ______________ Calculate the sample standard deviation sx number of cars per five minute period.

Presume that the number of cars per five minute period distribution data comes from a normal distribution. The following problems derive from chapter seven. Use the standard normal distribution to determine your answers. Use the sample mean x from question one for µ, and the sample standard deviation sx from question two for σ in the questions below. Use a spreadsheet to calculate z values and probabilities as appropriate. Here the variable is x and NOT x.
normal curve

  1. p(x ≥ 9) = ______________ Based on the sample above and the normal distribution, what percentage of five minute periods contained nine or more cars?
  2. p(x ≤ 3.9010) = ______________ Based on the sample above and the normal distribution, what percentage of five minute periods contained 3.9010 cars or less?
  3. p(x ≥ 15) = ______________ Based on the sample above and the normal distribution, what percentage of five minute periods contained 15 cars or more?
  4. p(x ≤ ______________) = 0.25 What is the number of cars below which are found the lowest 25% of the five minute periods?
Chapter nine: Confidence interval calculations

Use the sample mean x from question one and the sample standard deviation sx from question two and the data given in the first table to calculate a 95% confidence interval for the population mean µ number of cars per five minute periods using the student's t-distribution.

  1. sample size: n = ______________
  2. confidence level: c = ______________
  3. degrees of freedom: = ______________
  4. t-critical: tc = ______________
  5. Error tolerance E: = ______________
  6. Calculate the 95% confidence interval for the population mean µ number of cars per five minute period:

    P( ___________ ≤ µ ≤ ___________ ) = 0.95
  7. _________ Sixty cars passing a point in five minutes would classify a road as a major thoroughfare. Does the population mean include 60 cars in five minutes?
  8. _________ Six cars passing a point in five minutes would classify a road as a country lane. Does the population mean include 6 cars in five minutes?

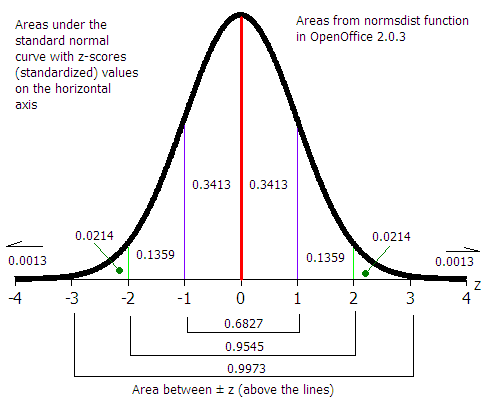
Standard normal distribution Excel: Left to z

Statistic or Parameter Symbol Equations Excel
Chapter seven: Normal statistics
Calculate a z value from an x z = standardize.gif (905 bytes) =STANDARDIZE(x; µ;σ)
Calculate an x value from a z x = z σ + µ =z*σ+µ
Find a probability p from a z value     =NORMSDIST(z)
Find a z value from a probability p     =NORMSINV(p)
Chapter eight: Distribution of the sample mean x
Calculate a z-statistic from an x z 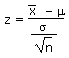xbartoz.gif (1022 bytes) =(x - µ)/(sx/SQRT(n))
Calculate a t-statistic (t-stat) t xbartot.gif (1028 bytes) =(x - µ)/(sx/SQRT(n))
Calculate an x from a z   xbarfromz.gif (1060 bytes) =µ + zc*sx/sqrt(n)
Chapter nine: Confidence interval statistics
Degrees of freedom df = n-1 =COUNT(data)-1
Find a tc value from a confidence level c and sample size n tc   =TINV(1-c;n-1)
Calculate an error tolerance E of a mean for any n ≥ 5 using sx. E error tolerance =tc*sx/SQRT(n)
Calculate a confidence interval for a population mean µ from the sample mean x and error tolerance E:   x-E ≤ µ ≤ x+E  

Calendar • Statistics • Lee Ling • COMFSM