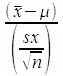
| Minutes |
|---|
| 2.77 |
| 2.58 |
| 2.68 |
| 2.45 |
| 2.47 |
| 2.48 |
| 2.52 |
| 2.52 |
| 2.59 |
| 2.76 |
| 2.56 |
| 2.71 |
| 2.84 |
| 2.65 |
| 2.90 |
| 2.78 |
| 2.78 |
| 2.73 |
| 2.74 |
| 2.42 |
On Wednesday evening 09 April 2008 I decided to go out and get some numbers for the Friday quiz. New numbers are always the best. Back in 2006 my mean joggling lap time was 2.55 minutes. Treat 2.55 as my pre-existing, previously known value. Use 2.55 minutes as my population mean μ lap time.
Wednesday evening I joggled twenty laps, with the times as given in the table. All times in the table are in minutes. The decimal is also in minutes. This is the sample. Could this sample come from a population with a mean μ of 2.55 minutes? That is the essence of this hypothesis test.
Use an alpha of α = 0.05 for the hypothesis test.
Null hypothesis: H0: μ = 2.55 minutes
Alternate hypothesis: H1: μ ≠ 2.55 minutes
| Hypothesis testing for a sample mean versus a known population mean | |||
|---|---|---|---|
| Statistic or Parameter | Symbol | Equations | OpenOffice |
| Relationship between confidence level c and alpha α for two-tailed tests | 1 − c = α | ||
| Calculate t-critical for a two-tailed test | tc | =TINV(α;df) | |
| Calculate a t-statistic | t | 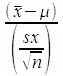 |
=(x - μ)/(sx/SQRT(n)) |
| Calculate a two-tailed p-value from a t-statistic | p-value | = TDIST(ABS(t);df;2) | |