Fibonacci, the Golden ratio, and Fibobelly

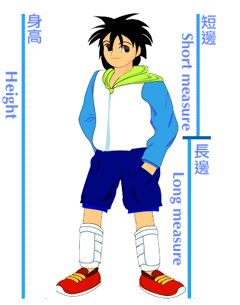
The site 15 Uncanny Examples of the Golden Ratio in Nature claims that "the measurement from the navel to the floor and the top of the head to the navel is the golden ratio." The golden ratio can be calculated from the expression shown on the right. $x=\frac{1+\sqrt{5}}{2}$ The expression generates an irrational number, a non-repeating decimal: 1.61803398874989484820458683436563811772030917980576... The site The Fibonacci Sequence- PROOF of GOD and that the World was Created/ Intelligently Designed claims that "From your toes to your belly button it is 1.618 times longer than your belly button to the top of your head." A mathematics web site makes the same claim, The Math Behind the Beauty argues that "Leonardo da Vinci's drawings of the human body emphasised its proportion. The ratio of the following distances is the Golden Ratio: (foot to navel) : (navel to head)". Is this true? These claims are that the long measure in the diagram below divided by the short measure in the diagram below is the golden ratio 1.618... We can test this claim by measuring our ratios and then calculating a confidence interval for the population mean based on our sample mean and sample standard deviation.

Calculate your FiboBelly ratio:

1. Short measure: __________ Measure the distance from your belly button to the top of your head.
2. Long measure: __________ Measure the distance from your belly button to the floor.
3. Ratio: __________ Long measure ÷ short measure
4. Put your FiboBelly ratio on the board!

Once all of the FiboBelly ratios are on the board, calculate the mean, standard deviation, and the standard error for the ratios.

5. sample size n: __________
6. sample mean x: __________
7. sample standard deviation sx: __________
8. sample standard error SE: __________ (the standard deviation divided by the square root of the sample size n)

An estimate of the confidence interval for the population mean that does not take into account the sample size can be obtained from the following calculation:

9. 2 * sample standard error SE: __________ Calculate 2 × the standard error of the mean
10. x − 2SE: __________
11. x + 2SE: __________
12. Construct the confidence interval for the fibobelly ratio population mean μ.

p(__________ ≤ population mean μ ≤ __________) < 0.95
  mean - 2SE                       mean + 2SE

Does the confidence interval for the mean fibobelly ratio include the golden ratio cited above?

An estimate of the confidence interval for the population mean that DOES take into account the sample size can be obtained from the following calculation:

13. tinv(1-0.95,n-1) * sample standard error SE: __________ Calculate t_critical × the standard error of the mean to obtain the margin of error E of the mean
14. x − 2E: __________
15. x + 2E: __________
16. Construct the 95% confidence interval for the fibobelly ratio population mean μ.

p(__________ ≤ population mean μ ≤ __________) = 0.95
  mean - tc*SE                     mean + tc*SE

Does the 95% confidence interval for the mean fibobelly ratio include the golden ratio cited above?